Descartes’ rule of signs is a fresh rule use to find the number of positive real zeros of a polynomial function. A mathematical technique called Descartes’ rule of signs can used to estimate how many positive and negative roots a polynomial equation with real coefficients could have. It bears the name of René Descartes, a French mathematician and philosopher who created this rule in the seventeenth century.
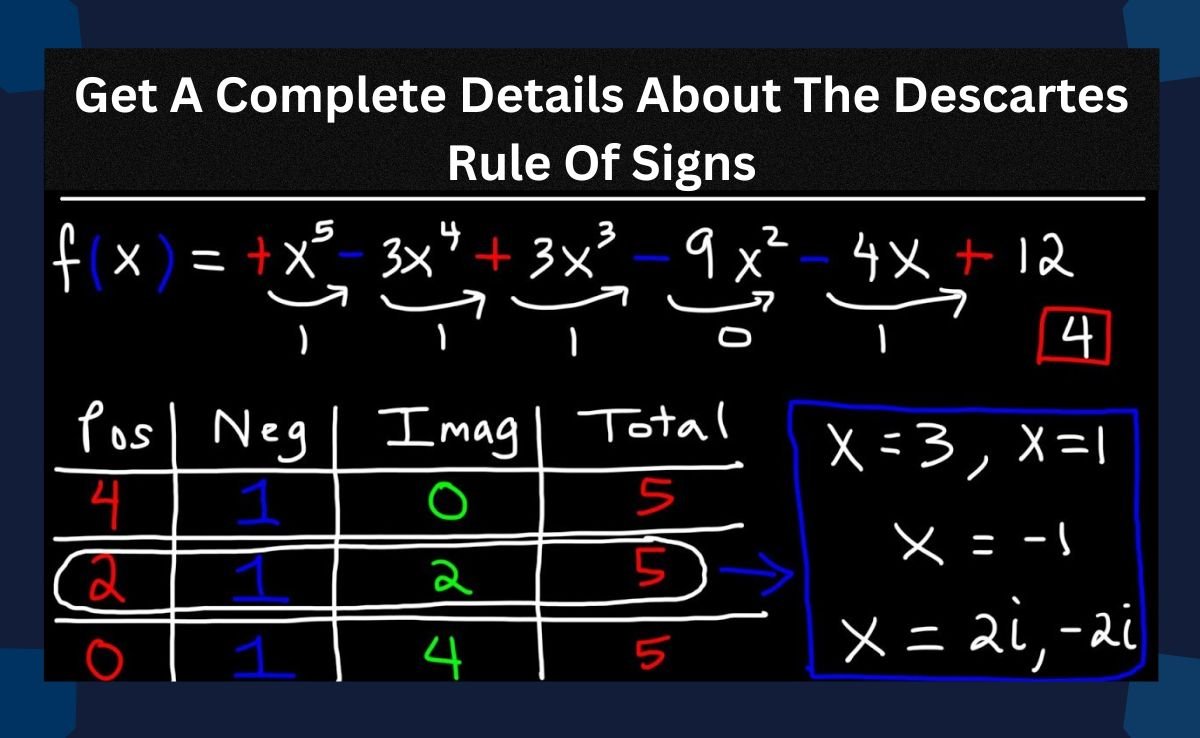
The quantity of positive, genuine foundations of a polynomial with genuine coefficients is equivalent to the number of sign changes in the coefficients or less by a much number, as per Descartes’ standard of signs. Then again, the quantity of negative genuine roots is equivalent to the quantity of sign changes in the coefficients of the polynomial f(- x) or less by a much number.
Here descartes rule of signs can utilized to track down the quantity of genuine zeros for a polynomial capability. In addition, if we repeat the procedure for the coefficients of the equation with various signs, that count will provide us with the maximum number of negative roots for the equation. Yet, it doesn’t furnish us with the exact number or upsides of the positive and negative roots, simply the greatest that can exist. It indicates that counting the instances in which the coefficients’ signs shift from left to right will yield the greatest number of positive roots for the equation.
State Descartes Rule of Sign
Let f(x)=anxn+an−1xn−1+…+a0 be a polynomial with real coefficients. After eliminating the terms with a value of 0 from the sequence an,an−1,….a0, determine the number of terms in the remaining sequence that follow each other and have opposite signs, denoted by s. It provides us with the total number of sequence sign changes.
Positive roots:
The standard expresses that the positive underlying foundations of a solitary variable polynomial with genuine coefficients and nonzero terms organized by plunging variable example should either rise to or be not exactly the quantity of sign changes between its back to back coefficients.
A foundation of k is comparable to k roots.
In particular, the number of positive roots is equal to the number of sign changes if there are zero or one sign changes.
Negative root:
The number of sign changes that occur after multiply the coefficients of odd-power terms one, or less than it an even integer, is known as the number of negative roots. It is the rule’s corollary. This procedure is equivalent to reverting the original variable to its negation. Consequently, when Descartes’ rule of signs is applied to this polynomial, it has the maximum number of negative roots that can exist.
Descartes’ rule of signs does not provide an exact number of roots; it only provides the number of positive as well as negative real roots that are possible. Consequently, we are able to create a chart that shows the potential number of positive, real, and imaginary roots. The following considerations must made while creating this chart.
In the previous example, we learned how to determine the maximum number of positive and negative real roots that a polynomial function can have. In this manner, the number of genuine roots, whether positive or negative, is either not exactly the number of sign changes by a considerable number or equivalent to the number of sign changes of f(x) (or f(- x)).
Keep in mind that the actual number of positive or negative real roots is the one that cannot reduced further by an even number if the number of real roots is 0 or 1. In this manner, don’t transform it across the outline, assuming we get 0 or 1 in any segment, positive or negative.
Besides, observe that the level of a polynomial capability is equivalent to the quantity of roots. In this way, the amount of positive and genuine roots can deducted from the polynomial’s certificate to decide the quantity of complicated roots.
The following describes how to apply Descartes’ Rule of Signs.
Step 1: Analyze the polynomial’s values for each term. It is possible to monitor a change in sign by being aware of the signs of the coefficients.
Step 2 : To find the total number of real roots, create an algebraic equation in the form P(x) for the positive real roots and P(-x) for the negative real roots.
Step 3: It is important to keep an eye out for indicative sign changes, which can happen a sign changes positive to negative, negative to positive, at all. There has been a sign shift if the two signs of adjacent coefficients change.
Step: 4 Count the different combinations of signs. If n is the number of sign variants, then number of positive and negative real roots could equal n, n-2, n-4, n-6, so on. Remember to subtract it by two every time. Stop subtracting as soon as the difference approaches zero or one.
Conclusion:
Descartes’ standard of sign is utilize to decide the number of genuine zeros there that are in a polynomial capability. Descartes rule of signs express that since there have two sign changes; there are either zero or two positive roots. It shows that quantity of coefficient sign changes in polynomial capability f(x) is equivalent to not exactly a large number.